Electric dance: a Coulombian 3-body problem with strong symmetries
I’m working on a simulation about a 3-body Coulombian system with very strong symmetries.
You can download the approved Mathematica code and/or the Wolfram CDF file at Wolfram Demonstration Project.
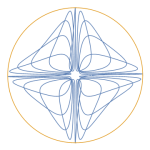
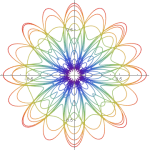
Three charged particles, two positive (blue) and one negative (red) are released from rest at the vertices of an isosceles triangle (equilateral in the initial setting).
It’s assumed that the particles have the same charge (but for the sign), the same mass (inertia) and that only the electric force acts on them.
The system dynamics will be just driven by Coulombian attractive/repulsive forces.
Anyway, given the strong symmetries in the initial conditions and given the conservation of energy and momentum, the system can be reduced to just a couple of differential equations, since the position/velocity of one of the blue particles is enough to set the positions/velocities of the other two. Here’s a video of the resulting “Electric dance”:
More info on this related page
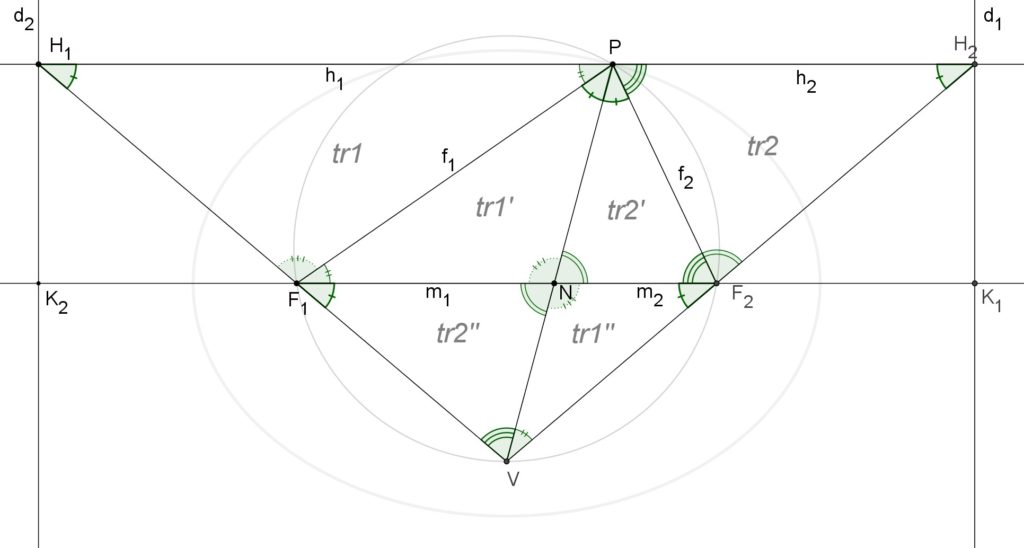 read more…
read more…