Sometimes math can be completely useless, but amazingly simple and beautiful.
A possible example of this is the following image, that could be created with a twitter-sized code in Wolfram Mathematica (123 characters in the present case):

Graphics3D[Table[Rotate[Cuboid[{-0.9^k, -0.9^k, (1/20)*k}, 
{0.9^k, 0.9^k, (1/20)*(k + 1)}], k*0.1, {0, 0, 1}], {k, 0, 60}]]

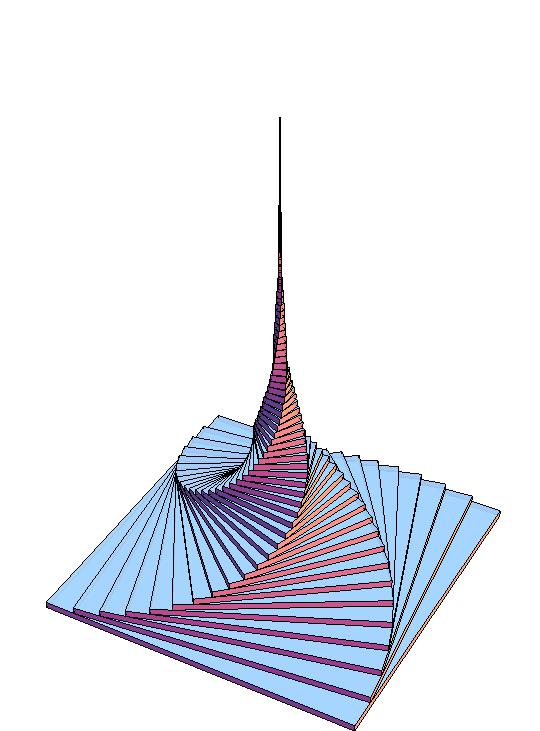This mini-program was published (and favorited) in the twitter @wolframtap (Wolfram Tweet-a-Program).

Here‘s the twit.

Another interesting thing about the fancy building depicted in the image is that, although it might have infinite height, it’ll still have a finite volume.

There’s also a small extension in this interactive demonstration (in which it’s possible to change the angle between consecutive parallelepipeds.

(Thanks to BV for suggesting me this beautiful idea)